Numbers

In this first article of our topic ‘Numbers’, we deal with ‘Numbers’ themselves.

So, what are numbers? Where do we see and meet them? Are they of different types? Can we learn these distinct types of numbers?

Well, numbers are all around us, floating all over the place. They demand our attention and careful evaluation. A number here or there can make a huge difference. In fact, a misplaced zero or decimal is the difference between one being poor or rich.

Let us start from types of numbers :-

Natural numbers: Natural numbers are the numbers which are used in counting . These numbers start from 1 and are followed by 2,3 …… so on .

Whole Numbers: Whole numbers include all natural numbers and “ZERO” .

Therefore, we can say,

Natural numbers + Zero = whole numbers

It means that every natural number is a whole number but the converse is not true.

Integers: Integers is the set of numbers which include whole numbers as well as their negatives. So

{0, – 1, 1, -2, 2, -3, 3…….} all are integers.

We can say that every whole number is an integer but the converse is not true.

Integers are further categorised into 2 sub-parts- Positive integers and negative integers.

Positive integers include {1, 2, 3…….}

Negative integers include {-1, -2, -3…….}

Also, if we add “zero” to the set of positive integers, it is called non-negative integers. They can be expressed as {0,1, 2, 3…….}

If we add “zero” to the set of negative integers, it is called non-positive integers. They can be expressed as {0,-1, -2, -3…….}

Rational numbers: Rational numbers are the numbers which can be written in form , where .

is a mandatory condition ,because if q becomes zero the number will not be defined.

Now let us understand rational numbers more closely.

What is p/q? Have you heard of fractions?

The numbers that are in the form: are called fractions.

You could also notice that the numbers you just read above( natural numbers, whole numbers, integers, etc.) can also be represented in form.

For example,

Natural numbers like ( 1,2,3…) ,whole numbers (0, 1,2,3…) OR negative numbers (-1,-2,-3 ….) can be represented as

Hence, every integer is a rational number but again the converse is not true.

Justifying this with an example, it can be observed that 2 is an integer and can be expressed as a rational number in the form of 2/1.However, ½ is a rational number but not an integer.

In addition to these numbers, rational numbers can also be expressed in the following two ways:

(a) All terminating decimals are rational numbers. e.g. 0.125 = 125/1000 = 1/8

(b) Non terminating recurring decimals are also rational numbers. e.g. 0.343434……, 1.25252525… These numbers can be expressed as the rational numbers.

Let us see how :-

Q1 . Express in the form of a fraction .

Solution.:

We know that =0.7777

Let x = 0.7777 …… (1)

Multiply the given equation by 10

10x= 7.77 …… (2)

Subtract (1) from (2) we get

10x- x=7.77 -0.77

9x = 7

X =7/9

This way you can convert other recurring decimals into fraction form. Hence, you can see that is a rational number.

We have added some questions to exercise at the end of this lesson for your practice.
Let us quickly sum up what we have read :-

Number type	RANGE
Natural numbers	{1 ,2,3,4 ,5 ……..}
Whole numbers	{0,1,2,3,4,5………}
Integers	All above numbers and their negatives:- {-4,-3,-2,-1,0,1,2,3,4,5………}
Positive Integers	{1,2,3,4,5………}
Negative Integers	{…., -4,-3,-2,-1)
Non Positive Integers	{…., -4,-3,-2,-1, 0)
Non Negative Integers	{0,1,2,3,4,5………}
Fractional numbers	{ ………….}
Rational numbers	Integers and fractional numbers

Irrational numbers: Contrary to Rational numbers there are numbers which cannot be written in form, these are called Irrational numbers.

Hence, non terminating non recurring decimals are called irrational numbers.

This includes

CAUTION :- Perfect square numbers are not irrational .

For e.g. . so check twice before marking your answer.

Prime numbers: The natural numbers, which have exactly two factors, 1 and the number itself, are called prime numbers.

For example 2, 3, 5, 7, 11, 13, 17, 19,……………… are prime numbers. We can say that the numbers which are not divisible by any number except one and itself, are called prime numbers.

There are 25 prime numbers between 1 and 100. They are as follows: 2, 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37, 41, 43, 47, 53, 59, 61, 67, 71, 73, 79, 83, 89, 97.

Composite numbers: The composite numbers are those natural numbers which can be written as the product of prime numbers in a unique way.

Or we can say that the number which is the product of two or more than two distinct or same prime numbers is said to be a composite number.

Example 4, 6, 8,…

Note that composite number always has more than two factors.

Note: 1 is neither prime nor composite.

REAL NUMBERS :- All the numbers you have read above are real numbers . It includes all rational and irrational numbers .

Any number which can be plotted on the number line is a real number.

A number line, what is that?

Number line : A number line is line where all the numbers are allocated their positions. The origin of the number line starts from zero and it continues to infinity, on either side.

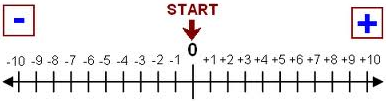
We have studied about integers, integers are combination of positive whole numbers and their negatives lying on the number line. There are few important properties regarding integers that you should know:

Zero is also an Integer.
Integers which follow one another are called consecutive integers.
If we multiply a number by an integer and resultant value remains same then that integer is 1.

Consecutive Integers: The integers which follow one another are called consecutive integers.

For example 3, 4, 5, 6 are consecutive integers. An individual random number can never be

a consecutive integer.

Note: If we have consecutive integers in a set M from a to b i.e. M ={a,a+1,a+2,……………………..,b}.

Then number of elements in the set M is b–a+1.

Example: Set M consists of natural numbers from 75 to 199. Find the numbers of elements in set M ?

Solution: Question would have been very easy if we were asked number of natural numbers from 1 to 199. The answer to this would have obviously been 199.

Here also we can proceed it in the same way. From 75 to 199 we are not counting first 74 natural numbers. Hence, the answer should be 199 -74= 125 (or 199-75+1)

Some more points about Consecutive Numbers

TOOLTIP 1

If there are odd number of digits in the set of consecutive numbers like set of three consecutive numbers (4,5,6,) or 5 digits are there, say (3,4,5,6,7), then in this case the sum of all integers is always divisible by the number of digits present in the set.

For example 4 + 5 + 6 = 15 is divisible by number of digits i.e. 3.

For example 3+4+5 + 6 + 7 = 25 is divisible by 5.

TOOLTIP 2

On the other hand ,If there are even number of digits in set of consecutive numbers like set of four consecutive numbers (4,5,6,7) or 6 numbers, say (3,4,5,6,7,8), then in this case the sum of all integers is never divisible by the number of digits present in the set .

For example 4+5 + 6 + 7 = 22 is not divisible by number of digits i.e. 4

For example 3+4+5+6 + 7 + 8 = 33 is not divisible by 6.

Having understood the basics about numbers, let us test our learning by solving the given exercise.

EXERCISE :-

Q1. Choose whether the following numbers are Rational or irrational .

a. 7

b. 8