The world of Arithmetic is the world of practical maths. We see it all around: numbers, ratios, and percentages floating all around us. In this same world, we have something known by the name of ‘Profit and Loss’.
What is Profit and Loss all about in the world of Mathematics?
Well, go back to your childhood and recollect all those trips to the market where you used to work yourself up in a frenzy just to understand what the shopkeeper had done. You used every ounce of your mental strength to calculate prices, check totals and MRPs just to be sure that you were not cheated. That, my friend, was your first introduction with the world of Arithmetic: the world of cost-price, selling-price, mark-ups and discounts. We explore the very same world today, for your benefit.
As a starter, let us dig into basic Profit and Loss concepts. We begin with the terminology for this topic.
Tooltip-1: Profit and Loss Definitions
Cost Price: The price (amount) paid to purchase a product or the cost incurred in manufacturing a product is known as the cost price (CP) of that product.
Types of cost
1. Fixed cost: It is a type of cost which is fixed under all conditions and does not vary according to the number of units produced.
2. Variable cost: Variable cost is a type of cost which varies according to the number of units. This is quite easy to understand.
3. Semi-variable cost: As the name suggests, these costs are the ones that are fixed in part and variable in part. Effectively, this is the case that we see most often.Imagine the scenario in a factory. There is a capital cost, which remains the same under all conditions (fixed cost) and a variable cost of the product, which in turn depends upon various factors.
Selling Price: The price at which a product is sold is called the selling price (SP) of the product.
Marked Price: Do shopkeepers put up price on the label that they wish to sell on or they put up an inflated price? If you think closely, majority of the shopkeepers mark-up their products, in anticipation of the discounts they would have to offer. This is a clever way of operating. Mark-up the price in advance, offer a discount and make the customer feel happy, and then sell the product. Pretty effective, eh?
List Price: List price or the tag price is the price that is printed on the tag of the article. For all practical purposes, we assume it to be same as the marked-price.
Margin: The profit percentage on selling price is known as MARGIN.
Example 1: Pranav sell an article at a discount of 80% and get a profit of 60% on that article calculate the mark up over the cost price?
Solution:
Let us put the above definitions in use.
Let us assume that Cost Price = Rs 100.
So, Selling Price = Rs 160.
Now, after giving a discount of 80% over MP, Rs 160 is the SP.
Let the Marked Price: MP
SP = 20% of MP
160 = 20% of MP
MP = 800
% Mark Up = (700/100) × 100 = 700 %.
Things to remember in this question:
Mark Up over Cost Price = Marked Price – Cost Price = M
% Mark Up Cost Price = [{M/CP} x 100]
Mark Up over Selling Price = Marked Price – Selling Price = M
% Mark Up Selling Price = [{M/SP} x 100]
Focus closely on what is being asked. Remember Profit and Loss is nothing else but an application of Percentages and basic calculation skills.
Tooltip 2: Profit and Loss Basic Concepts
1. One can generate a profit only if Selling Price> Cost Price
2. One generates a loss when Selling Price < Cost Price.
3. Profit = Selling Price – Cost Price
% Profit = [{(Selling Price -Cost Price)/Cost Price} x 100]
4. Loss = Cost Price – Selling Price
% Loss = [{(Cost Price-Selling Price)/Cost Price} x 100]
Tooltip-3: Profit and Loss Multiplying equivalents to figure out the sale price and cost price
Equivalent for Sale Price:
If there is a profit of P %,
Cost Price = CP
Then, SP = {(100+P)/100} x CP
If there is a loss of L %,
Cost Price = CP
Then SP = {(100-L)/100} x CP
Equivalent for Cost Price:
If there is a profit of P %,
Cost Price = CP
Sale price= SP
Then,
CP = [{100/(100+P)} x SP]
If there is a loss of L %,
Then
CP =[{100/(100+L)} x SP]
Example-1: Mehak and Pranav sells some article for Rs 8000 each. Mehak calculates her profit per cent on his CP and Pranav calculates his profit per cent wrongly on SP. What is the difference in their actual profit if both claim to have a profit of 60%?
Solution:
For Mehak
SP = Rs 8,000
Profit = 60% of CP
CP = Rs 5000
Profit = Rs 3000
For Pranav
SP = Rs 8,000
Profit = 60% of SP = 60% of 8000 = 4800
CP = Rs 3,200
Profit = Rs 4800
So, the difference in profit = Rs 1800
Example-2: Megha and Richa sold two articles at Rs 12,000 each. One is sold at a profit of 20% and another one at a loss of 20%. What is the net loss?
Solution:
For article-1
SP 1 = Rs 12,000
CP1 = Rs 12,000/1.2 = Rs 10,000
For article-2
SP2 = Rs 12,000
CP2 = Rs 12,000/0.8 = Rs 15,000
So, total CP = Rs 25,000 and total SP = Rs 24,000
So, loss = Rs 1,000.
Profit and Loss: Basic Concepts Exercise
Question 1: If an article is sold at 5% gain instead of 5% loss, the man gains Rs. 5 more. Find the cost price of that article
(1) Rs. 100
(2) Rs. 105
(3) Rs. 50
(4) Rs. 110
Answer and Explanation
Let the cost price be p
On 5% gain, the selling price is = [p x {(100+5)/100}]= 1.05p
On 5% loss, the selling price is =[p x {(100-5)/100}]= 0.95
The question says that if an article is sold at 5% gain instead of 5% loss, the man gains Rs. 5 more so
1.05p – 0.95p = 5
=> 0.1p = 5
=> p = Rs 50
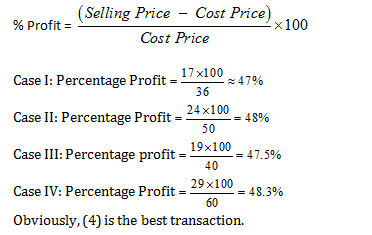
Question 2: In terms of percentage profit, which is the best transaction?
C.P. (in Rs.) Profit (In Rs.)
Transaction (I) 36 17
Transaction(II) 50 24
Transaction(III) 40 19
Transaction(IV) 60 29
(1) I
(2) II
(3) III
(4) IV
Answer and Explanation
Question 3: A merchant buys an article for Rs. 27 and sells it at a profit of 10% of the selling price. The sell¬ing price of the article is:
(1) Rs. 29.70
(2) Rs. 30
(3) Rs. 37
(4) Rs. 32
Answer and Explanation
Option : (2)
CP= Cost Price
SP= Selling Price
Here we have S.P. – C.P. = (10S.P.)/100 = (S.P./10)
{S.P. –(S.P./10)} = C.P.
{SP x (9/10)} = CP
As CP = Rs. 27, we get
= S.P. = (27 x 10)/9 = Rs. 30
Question 4: Krishnan bought a camera and paid 20% less than its original price. He sold it at 40% profit on the price he had paid. The percentage of profit earned by Krishnan on the original price was
(1) 22%
(2) 32%
(3) 12%
(4) 15%
Answer and Explanation
Solution: (3)
CP= Cost Price
SP= Selling Price
Let the original price be Rs. p.
CP = (80/100)p=Rs. 4p/5
SP = (4P/5)x(140/100) = Rs. 28p/25
Gain on original price=sp-cp = 28p/25 – p = 3p/25
Therefore Gain % = (Gain/ Original Price ) x 100
Gain Percentage = {Gain /(Original Price ) x 100}
Gain Percentage = (3p/25p) x 100 = 12%
Question 5: By selling a basket for Rs. 19.50, a shopkeeper gains 30%. For how much should he sell it to gain 40%?
(1) Rs. 21
(2) Rs. 21.50
(3) Rs. 24
(4) Rs. 23
Answer and Explanation
Solution: (1)
CP= Cost Price
SP= Selling Price
CP = {100/(100+P)} x SP
CP = {(19.50×100)/130}= Rs. 15
To gain 40% profit
Therefore SP = {CPx(100+profit)/100}
SP = (140 x 15)/100 = Rs. 21







