- This is an assessment test.
- These tests focus on the basics of Maths and are meant to indicate your preparation level for the subject.
- Kindly take the tests in this series with a pre-defined schedule.
Basic Maths: Test 29
Congratulations - you have completed Basic Maths: Test 29.You scored %%SCORE%% out of %%TOTAL%%.You correct answer percentage: %%PERCENTAGE%% .Your performance has been rated as %%RATING%%
Your answers are highlighted below.
Question 1 |
Given that 12+22+32+….+202=2870, the value of (32+62+92+….+602) is :
11480 | |
5740 | |
25830 | |
2870 |
Question 1 Explanation:
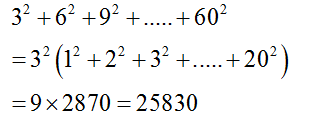
Question 2 |
13+23+33+…… +103=3025 and 13+23+33+43+53=225, then 63+73+83+93is equal to
1800 | |
1705 | |
1025 | |
2420 |
Question 2 Explanation:
$ \displaystyle \begin{array}{l}{{1}^{3}}+{{2}^{3}}+{{3}^{3}}+....+{{10}^{3}}=3025\\\And {{1}^{3}}+{{2}^{3}}+{{3}^{3}}+{{4}^{3}}+{{5}^{3}}=225\\now,\\{{6}^{3}}+{{7}^{3}}+{{8}^{3}}+{{9}^{3}}\\={{1}^{3}}+{{2}^{3}}+{{3}^{3}}+.....+{{10}^{3}}-({{1}^{3}}+{{2}^{3}}+{{3}^{3}}+{{4}^{3}}+{{5}^{3}})-{{10}^{3}}\\=3025-225-1000\\=1800\end{array}$
Question 3 |
(25+26+27+…..+93+94+95) is equal to
5260 | |
5660 | |
4260 | |
4000 |
Question 3 Explanation:
$ \begin{array}{l}\left( 25+26+27+.....+94+95 \right)\\=\left( 1+2+3+.....+95 \right)-\left( 1+2+3+.....+24 \right)\\=\frac{95\times \left( 95+1 \right)}{2}-\frac{24\times \left( 24+1 \right)}{2}\\\left[ 1+2+3+....+n=\frac{n\left( n+1 \right)}{2} \right]\\=\frac{95\times 96}{2}-\frac{24\times 25}{2}\\=95\times 48-12\times 25\\=4560-300=4260\end{array}$
Question 4 |
$ \frac{2}{3}+\frac{4}{60}+\frac{2}{35}+\frac{4}{126}+\frac{2}{99}$
$ \frac{10}{11}$ | |
$ \frac{1}{10}$ | |
$ \frac{11}{12}$ | |
$ \frac{11}{10}$ |
Question 4 Explanation:
Expression
$ \begin{array}{l}=\frac{2}{3}+\frac{4}{60}+\frac{2}{35}+\frac{4}{126}+\frac{2}{99}\\=\frac{2}{3}+\frac{2}{15}+\frac{2}{35}+\frac{2}{63}+\frac{2}{99}=1-\frac{1}{3}+\frac{1}{3}-\frac{1}{5}+\frac{1}{5}-\frac{1}{7}+\frac{1}{7}-\frac{1}{9}+\frac{1}{9}-\frac{1}{11}\\=1-\frac{1}{11}=\frac{11-1}{11}=\frac{10}{11}\end{array}$
$ \begin{array}{l}=\frac{2}{3}+\frac{4}{60}+\frac{2}{35}+\frac{4}{126}+\frac{2}{99}\\=\frac{2}{3}+\frac{2}{15}+\frac{2}{35}+\frac{2}{63}+\frac{2}{99}=1-\frac{1}{3}+\frac{1}{3}-\frac{1}{5}+\frac{1}{5}-\frac{1}{7}+\frac{1}{7}-\frac{1}{9}+\frac{1}{9}-\frac{1}{11}\\=1-\frac{1}{11}=\frac{11-1}{11}=\frac{10}{11}\end{array}$
Question 5 |
$ \displaystyle \begin{array}{l}\sqrt{17}=4.123\,approximately\\\sqrt{170\,}=13.04\text{ }\left( approximately \right)\\Find\,\,the\,\,value\,\,of\\\sqrt{1.7}+\sqrt{1700}+\sqrt{00.17}\end{array}$
41.9463 | |
43.9463 | |
42.9463 | |
40.9463 |
Question 5 Explanation:
$ \displaystyle \sqrt{1.7}+\sqrt{1700}+\sqrt{00.17}$
$ \begin{array}{l}=\sqrt{\frac{170}{100}\,}+10\sqrt{17}+\sqrt{\frac{17}{100}}\\=\frac{1}{10}\sqrt{170}+10\sqrt{17}+\frac{1}{10}\sqrt{17}\\=\frac{13.04}{10}+4.123\times 10+\frac{4.123}{10}\\=1.304+41.23+0.4123\\=42.9463\end{array}$
$ \begin{array}{l}=\sqrt{\frac{170}{100}\,}+10\sqrt{17}+\sqrt{\frac{17}{100}}\\=\frac{1}{10}\sqrt{170}+10\sqrt{17}+\frac{1}{10}\sqrt{17}\\=\frac{13.04}{10}+4.123\times 10+\frac{4.123}{10}\\=1.304+41.23+0.4123\\=42.9463\end{array}$
Once you are finished, click the button below. Any items you have not completed will be marked incorrect.
There are 5 questions to complete.
List |











