- This is an assessment test.
- These tests focus on the basics of Maths and are meant to indicate your preparation level for the subject.
- Kindly take the tests in this series with a pre-defined schedule.
Basic Maths: Test 3
Congratulations - you have completed Basic Maths: Test 3.You scored %%SCORE%% out of %%TOTAL%%.You correct answer percentage: %%PERCENTAGE%% .Your performance has been rated as %%RATING%%
Your answers are highlighted below.
Question 1 |

$ \displaystyle \frac{123}{122}$ | |
$ \displaystyle \frac{145}{154}$ | |
$ \frac{205}{146}$ | |
$ \displaystyle \frac{147}{157}$ |
Question 1 Explanation:
We do solve the first half and then we solve the whole question

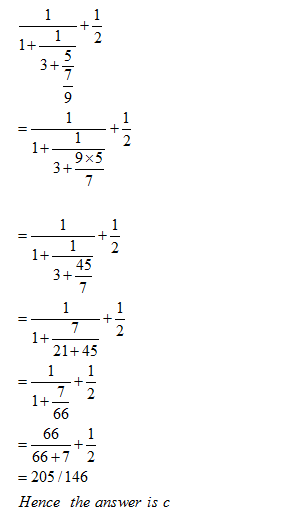
Question 2 |
If $ \displaystyle x=1+\frac{1}{2+\frac{1}{3+\frac{1}{4+\frac{1}{2}}}}$
Then, the value of $ \displaystyle 2x+\frac{1}{2}$ is
$ \displaystyle 3\frac{49}{134}$ | |
$ \displaystyle 5\frac{13}{24}$ | |
$ \displaystyle 7\frac{13}{24}$ | |
$ \displaystyle 6\frac{23}{134}$ |
Question 2 Explanation:
In this we need the value of x so for value of x we will solve the complex fraction part first .
$ \displaystyle \begin{array}{l}x=1+\frac{1}{2+\frac{1}{3+\frac{1}{4+\frac{1}{2}}}}\\x=1+\frac{1}{2+\frac{1}{3+\frac{2}{9}}}\\x=1+\frac{1}{2+\frac{9}{29}}\\x=1+\frac{29}{67}\\x=96/67\\Now\,by\,\,filling\,\,the\,\,value\,of\,\,x\\we\,\,have\,\,\\2x+\frac{1}{2}=2\times 96/67+\frac{1}{2}\\=\,451/134\,\,=\,\,3\frac{49}{134}\end{array}$
$ \displaystyle \begin{array}{l}x=1+\frac{1}{2+\frac{1}{3+\frac{1}{4+\frac{1}{2}}}}\\x=1+\frac{1}{2+\frac{1}{3+\frac{2}{9}}}\\x=1+\frac{1}{2+\frac{9}{29}}\\x=1+\frac{29}{67}\\x=96/67\\Now\,by\,\,filling\,\,the\,\,value\,of\,\,x\\we\,\,have\,\,\\2x+\frac{1}{2}=2\times 96/67+\frac{1}{2}\\=\,451/134\,\,=\,\,3\frac{49}{134}\end{array}$
Question 3 |
$ \displaystyle 16\times 54\div 18=a$
50 | |
52 | |
44 | |
None |
Question 3 Explanation:
We can simplified the equation as
$ \displaystyle a=16\times \frac{54}{18}$ So the value of a will be 48 hence d is the right answer
$ \displaystyle a=16\times \frac{54}{18}$ So the value of a will be 48 hence d is the right answer
Question 4 |
Find the value of m in
$ \displaystyle 785-m+12\times 11=6295$
-5883 | |
-5793 | |
-5378 | |
-5563 |
Question 4 Explanation:
The equation can be simplified to
$ \displaystyle \begin{array}{l}785-m+12\times 11=6295\\or,\,\,6295+m=785+12\times 11\\m=785+12\times 11-6295\\m=\text{-5378}\end{array}$
Therefore the right answer is option c
$ \displaystyle \begin{array}{l}785-m+12\times 11=6295\\or,\,\,6295+m=785+12\times 11\\m=785+12\times 11-6295\\m=\text{-5378}\end{array}$
Therefore the right answer is option c
Question 5 |
If
$ \displaystyle {{9}^{5.5}}\times {{9}^{y}}={{9}^{9}}$
then find the value of y
$ \displaystyle {{9}^{5.5}}\times {{9}^{y}}={{9}^{9}}$
then find the value of y
4.5 | |
2.29 | |
2.75 | |
none |
Question 5 Explanation:
In case of multiplication if bases are same then the power can be added
So here 5.5 + y =9
From here y = 9 - 5.5 = 3.5
Hence the option d is the right answer
So here 5.5 + y =9
From here y = 9 - 5.5 = 3.5
Hence the option d is the right answer
Once you are finished, click the button below. Any items you have not completed will be marked incorrect.
There are 5 questions to complete.
List |












i think u ve wrong answer of this question no 1………………………………it should be 139/146
Hey Prabhat, check your calculations. The answer seems to be correct in this case. You can post your solution as an image.
sorry made a calculation mistake:P ..its correct
The Answer figure is wrong it should be :
m=785+12×11−6295
hence, m=-5368