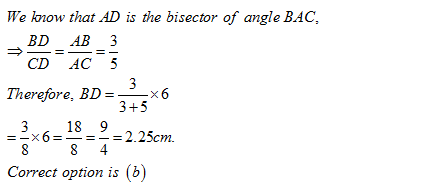Geometry and Mensuration: Test 17

This is an assessment test.
These tests focus on geometry and mensuration and are meant to indicate your preparation level for the subject.
Kindly take the tests in this series with a pre-defined schedule.

Geometry and Mensuration: Test 17

Please wait while the activity loads.
If this activity does not load, try refreshing your browser. Also, this page requires javascript. Please visit using a browser with javascript enabled.

If loading fails, click here to try again

Congratulations - you have completed Geometry and Mensuration: Test 17.You scored %%SCORE%% out of %%TOTAL%%.You correct answer percentage: %%PERCENTAGE%% .Your performance has been rated as %%RATING%%

Your answers are highlighted below.

Question 1

In a triangle ABC, the lengths of the sides AB, AC and BC are 3, 5 and 6 cm respectively. If a point D on BC is drawn such that the line AD bisects the ∠A internally, then what is the length of BD?

A	2 cm
B	2.25 cm
C	2.5 cm
D	3 cm

Question 1 Explanation:

geometry-and-mensuration-test-17-question-1-pic-1

Question 2

If P and Q are the mid-points of the sides CA and CD respectively of a triangle ABC right angled at C. Then, the value of 4 (AQ² + BP²) is equal to

A	4 BC²
B	5 AB²
C	2AC²
D	2BC²

Question 2 Explanation:

$ \displaystyle \begin{array}{l}A{{D}^{2}}=\text{ }A{{C}^{2}}+\text{ }C{{D}^{2}}\\In\,\,PCB,\\\begin{array}{*{35}{l}} B{{P}^{2}}=\text{ }P{{C}^{2}}+\text{ }C{{B}^{2}} \\ A{{D}^{2}}+B{{P}^{2}}=\text{ }A{{C}^{2}}+C{{D}^{2}}+P{{C}^{2}}+\text{ }C{{B}^{2}} \\ =\text{ }\left( A{{C}^{2}}+C{{B}^{2}} \right)\text{ }+\text{ }\left( C{{D}^{2}}+\text{ }P{{C}^{2}} \right) \\ =\text{ }A{{B}^{2}}=\text{ }P{{D}^{2}} \\ \end{array}\\=A{{B}^{2}}{{\left( \frac{1}{2}\,AB \right)}^{2}}\\A{{D}^{2}}+\text{ }B{{P}^{2}}=\frac{4A{{B}^{2}}+A{{B}^{2}}}{4}\\Therefore\text{ }4\text{ }\left( A{{D}^{2}}+\text{ }B{{P}^{2}} \right)\text{ }=\text{ }5A{{B}^{2}}\end{array}$

Question 3

If the sides of a right triangle are x,x+1 and x-1,then the hypotenuse is

A	5
B	4
C	1
D	0

Question 3 Explanation:

Since the triangle is a right angled triangle, We have, (x+1)² = x²+(x-1)²
=> x² +2x +1 = x² + x²-2x +1
=> x²- 4x =0
=> x(x-4) =0
=>x = 4 [x cannot be equal to 0 ]
Therefore Hypotenuse = 4+1 = 5

Question 4

Let ABC be an acute-angled triangle and CD be the altitude through C. If AB = 8 and CD= 6, then the distance between the mid-points of AD and BC is

A	30
B	25
C	27
D	5

Question 4 Explanation:

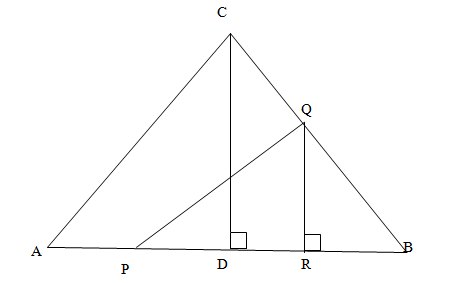
geometry-and-mensuration-test-17-question-4-pic-1

Let P and Q be the mid points of AD and BC respectively.
Draw QR perpendicular on AB. Since Q is the midpoint of BC, so R is the midpoint of BD. Hence by mid point theorem QR = 3.
Also AD = 4 = DB. So DR = PD = 2 Þ PR = 4. Hence by Pythagoras theorem, we have
PQ² = PR² + QR² = 16 + 9 = 25
=>PQ = 5.

Question 5

In ΔABC, ∠B is a right angle, AC =6 cm, and D is the mid-point of AC. The length of BD is: